
And we see that, at leastĪt that point, g of x is exactly 1 higher than that. Hope I didn't over explain, just proud of what I made tbh so 5*f(x) would make a point (2,3) into (2,15) and (5,7) would become (5,35)ī will shrink the graph by a factor of 1/b horizontally, so for f(5x) a point (5,7) would become (1,3) and (10,11) would become (2,11)Ĭ translates left if positive and right if negative so f(x-3) would make (4,6) into (7,6) and (6,9) into (9,9)ĭ translates up if positive and down if negative, so f(x)-8 would make the points (5,5) and (7,7) into (5,-3) and (7,-1)Īlso should note -a flips the graph around the x axis and -b flips the graph around the y axis. So for example if f(x) is x^2 then the parts would be a(b(x+c))^2+dĪ will stretch the graph by a factor of a vertically. Then if m is negative you can look at it as being flipped over the x axis OR the y axis.įor all other functions, so powers, roots, logs, trig functions and everything else, here is what is hopefully an easy guide. We can work around this by factoring inside the function.Yep, for linear functions of the form mx+b m will stretch or shrink the function (Or rotate depending on how you look at it) and b translates. This format ends up being very difficult to work with, because it is usually much easier to horizontally stretch or compress a graph before shifting. To solve for x, we would first subtract 3, resulting in a horizontal shift, and then divide by 2, causing a horizontal compression. What input to g would produce that output? In other words, what value of x will allow g\left(x\right)=f\left(2x+3\right)=12? We would need 2x+3=7. When we write g\left(x\right)=f\left(2x+3\right), for example, we have to think about how the inputs to the function g relate to the inputs to the function f. Horizontal transformations are a little trickier to think about. In other words, multiplication before addition. Given the output value of f\left(x\right), we first multiply by 2, causing the vertical stretch, and then add 3, causing the vertical shift. When we see an expression such as 2f\left(x\right)+3, which transformation should we start with? The answer here follows nicely from the order of operations. For example, vertically shifting by 3 and then vertically stretching by a factor of 2 does not create the same graph as vertically stretching by a factor of 2 and then vertically shifting by 3, because when we shift first, both the original function and the shift get stretched, while only the original function gets stretched when we stretch first. When combining transformations, it is very important to consider the order of the transformations. This means that the input values must be four times larger to produce the same result, requiring the input to be larger, causing the horizontal stretching. Our new population, R, will progress in 1 hour the same amount as the original population P does in 2 hours, and in 2 hours, the new population R will progress as much as the original population P does in 4 hours.

Let’s let our original population be P and our new population be R.

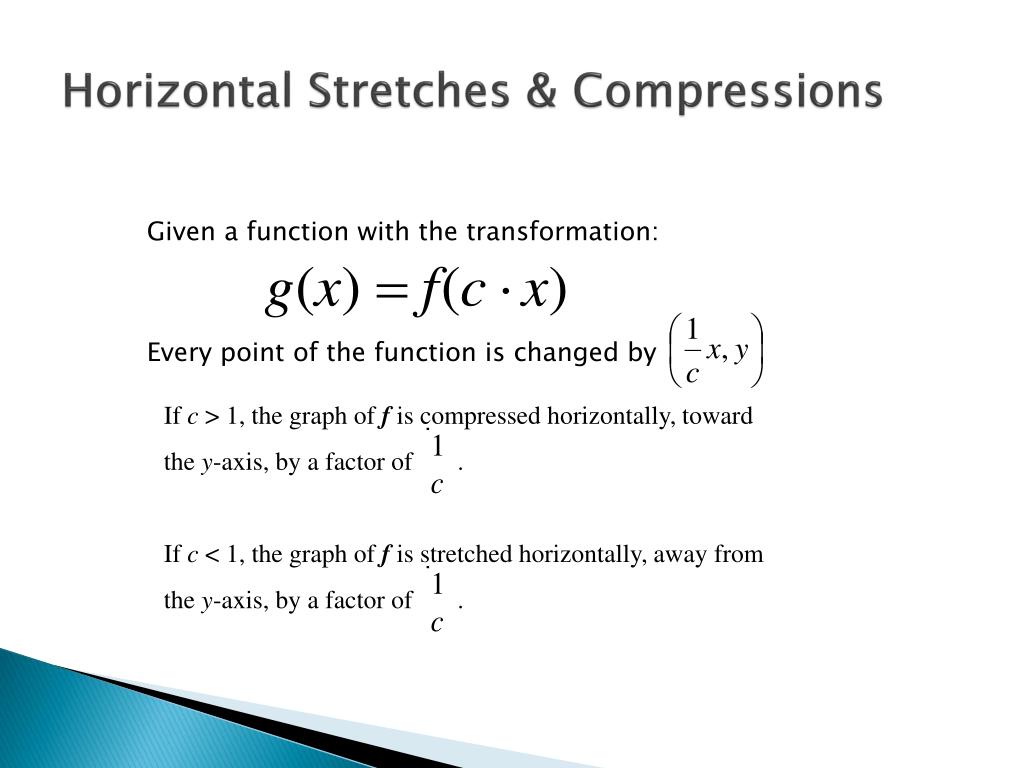
Suppose a scientist is comparing a population of fruit flies to a population that progresses through its lifespan twice as fast as the original population. If the constant is between 0 and 1, we get a horizontal stretch if the constant is greater than 1, we get a horizontal compression of the function. When we multiply a function’s input by a positive constant, we get a function whose graph is stretched horizontally away from or compressed horizontally toward the vertical axis in relation to the graph of the original function. Notice that we are changing the inside of a function. Now we consider the changes that occur to a function if we multiply the input of an original function f\left(x\right) by some constant.


 0 kommentar(er)
0 kommentar(er)
